Romberg integration algorithm using MATLAB

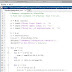
MATLAB PROGRAM:

% Romberg integration algorithm

 % Find the integral of y=sin(x) from 0 to pi.

 f = @(x) sin(x);

 a = input('Enter lower limit, a:  ');

 b = input('Enter upper limit, b:  ');

 n = input('Enter no. of subintervals, n:  ');

 

 h = b-a;

 r = zeros(2,n+1);

 r(1,1) = (f(a)+f(b))/2*h;

 fprintf('\nRomberg integration table:\n');

 fprintf('\n %11.8f\n\n', r(1,1));

 for i = 2:n

    sum = 0;

    for k = 1:2^(i-2)

       sum = sum+f(a+(k-0.5)*h);

    end

    r(2,1) = (r(1,1)+h*sum)/2;

  

    for j = 2:i

       l = 2^(2*(j-1));

       r(2,j) = r(2,j-1)+(r(2,j-1)-r(1,j-1))/(l-1);

    end

    for k = 1:i

       fprintf(' %11.8f',r(2,k));

    end

 

    fprintf('\n\n');

    h = h/2;

    for j = 1:i

       r(1,j) = r(2,j);

    end

 end

OutPut:

Enter lower limit, a:  0

Enter upper limit, b:  pi
Enter no. of subintervals, n:  6

Romberg integration table:

  0.00000000

  1.57079633  2.09439510

  1.89611890  2.00455975  1.99857073

  1.97423160  2.00026917  1.99998313  2.00000555

  1.99357034  2.00001659  1.99999975  2.00000002  1.99999999

  1.99839336  2.00000103  2.00000000  2.00000000  2.00000000  2.00000000


>>